In the realm of physics, the behavior of rotating bodies has always been a subject of fascination. This article, based on the insightful video “The Bizarre Behavior of Rotating Bodies” by Veritasium, delves into the intriguing phenomena known as the Dzhanibekov Effect or the Tennis Racket Theorem. This effect, while seemingly counterintuitive, offers a captivating insight into the world of rotational dynamics and its implications.

Contents

• The Dzhanibekov Effect
• The Tennis Racket Theorem
• Understanding the Intermediate Axis Theorem
• The Earth and the Dzhanibekov Effect
• The Role of Energy Dissipation in Rotation
• The Stability of Rotating Bodies: A Matter of Inertia
• The Role of Centrifugal Forces
• The Dzhanibekov Effect in Everyday Objects
• The Beauty of Physics
• The Mathematical Underpinnings
• A Bridge Between Classical and Modern Physics
• The Enduring Mystery
• The video
• References

The Dzhanibekov Effect

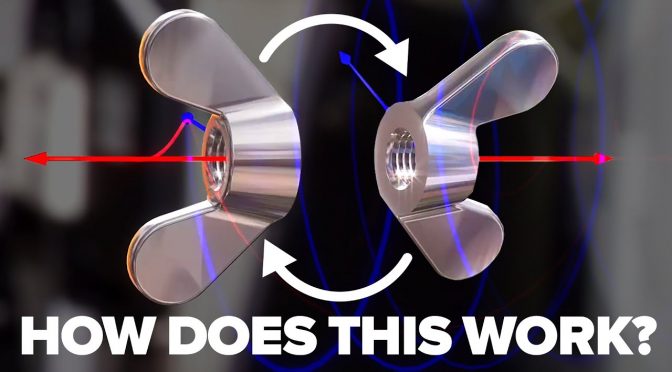
The Dzhanibekov Effect, named after the cosmonaut Vladimir Dzhanibekov, was first observed in space during a mission to save the Soviet space station Salyut 7 in 1985. Dzhanibekov noticed a peculiar behavior of a wing-nut as it spun off a bolt in the zero-gravity environment. The wing-nut maintained its orientation for a short time, then flipped 180 degrees, and continued this flipping at regular intervals. This motion wasn’t caused by any external forces or torques, making it a fascinating and mysterious phenomenon.

The Tennis Racket Theorem

The Dzhanibekov Effect is closely related to what is known as the Tennis Racket Theorem. This theorem states that if you flip a tennis racket in the air, it not only rotates the way you intend it to, but also makes a half turn around an axis that passes through its handle. This means that the side of the racket that was originally facing you will be facing away when you catch it. This theorem, like the Dzhanibekov Effect, is a manifestation of the intermediate axis theorem, which describes the rotation of three-dimensional objects.

Understanding the Intermediate Axis Theorem

The intermediate axis theorem, or the Dzhanibekov Effect, can be understood by considering an object with three different moments of inertia about its three principal axes. When an object is rotated about its first or third axis, the rotation is stable. However, when rotated about the second axis, the intermediate axis, the object exhibits a half twist, a behavior that is observed in the flipping of the tennis racket or the wing-nut in space.

The Earth and the Dzhanibekov Effect

The Dzhanibekov Effect has led to some speculation about whether the Earth, a spinning ball in space, could also flip over. However, this hypothesis is debunked by understanding that the Earth, like many astronomical objects, spins about the axis with the maximum moment of inertia, which is a stable rotation. This means that the Earth, contrary to some theories, will not flip over due to the Dzhanibekov Effect.

The Role of Energy Dissipation in Rotation

The behavior of rotating bodies can also be understood by considering energy dissipation. For an isolated object spinning in space, its angular momentum remains constant, but its kinetic energy can be converted into other forms of energy, like heat. This energy dissipation can cause the object to rotate about the axis that achieves the minimum kinetic energy, which is the axis with the maximum moment of inertia. This principle explains why objects like a liquid-filled cylinder or a satellite with flexible antennas end up rotating end-over-end.

The Stability of Rotating Bodies: A Matter of Inertia

The stability of a rotating body is largely determined by its moments of inertia, which are measures of the body’s resistance to changes in its rotation. The moments of inertia depend on both the mass distribution of the body and the axis about which it is rotating. For an object with three distinct principal axes, like a tennis racket or a wing-nut, it has three different moments of inertia.

When the object rotates about the axis with the smallest or largest moment of inertia, the rotation is stable. This is because the mass is distributed in such a way that it naturally resists changes in the rotation. However, when the object rotates about the intermediate axis, the rotation becomes unstable, leading to the unexpected flipping behavior observed in the Dzhanibekov Effect and the Tennis Racket Theorem.

The Role of Centrifugal Forces

Centrifugal forces, which appear when analyzing motion in a rotating frame of reference, play a crucial role in the Dzhanibekov Effect. When an object like a disc with point masses is rotating about the intermediate axis, the centrifugal forces act on the masses, pushing them away from the rotation axis. If the disc is bumped and starts rotating slightly off the intermediate axis, the centrifugal forces cause the masses to accelerate, leading to the flipping motion. This flipping continues at regular intervals, providing a visual demonstration of the Dzhanibekov Effect.

The Dzhanibekov Effect in Everyday Objects

The Dzhanibekov Effect is not limited to wing-nuts in space or tennis rackets. It can be observed in many everyday objects, as long as they have three different moments of inertia about their three principal axes. For example, a cell phone or a disc with a hole in it will exhibit the same flipping behavior when spun about the intermediate axis. This widespread occurrence of the Dzhanibekov Effect underscores its fundamental nature in the physics of rotating bodies.

The Beauty of Physics

The Dzhanibekov Effect, with its counterintuitive flipping behavior, serves as a testament to the beauty and complexity of physics. It challenges our intuition and invites us to delve deeper into the intricacies of rotational dynamics. By understanding this effect, we gain a deeper appreciation for the underlying principles that govern the motion of objects, from a tennis racket on Earth to a wing-nut in space. It is a reminder that even in the seemingly simple act of rotation, there are rich and fascinating phenomena waiting to be discovered.

The Mathematical Underpinnings

The Dzhanibekov Effect, while visually striking, is underpinned by complex mathematical principles. The intermediate axis theorem, which forms the basis of this effect, is a result of the Euler equations of motion for a rigid body (Landau and Lifschitz, 1976). These equations describe how the angular velocity of a body changes in response to a torque. When applied to a body rotating about the intermediate axis, they predict the unexpected flipping behavior observed in the Dzhanibekov Effect.

A Bridge Between Classical and Modern Physics

The Dzhanibekov Effect serves as a bridge between classical and modern physics. It is a classical phenomenon, rooted in the principles of rotational dynamics that have been known for centuries (Poinsot, 1851). Yet, it also has modern implications, as it was observed in the microgravity environment of space and has been studied using modern mathematical techniques (Tao, 2011). This dual nature of the Dzhanibekov Effect highlights the continuity and progression of scientific knowledge.

The Enduring Mystery

In conclusion, the Dzhanibekov Effect, with its mesmerizing flipping behavior, continues to captivate scientists and laypeople alike. It is a testament to the enduring mystery and beauty of physics, a field where even well-established principles can lead to surprising and counterintuitive phenomena. As we continue to explore the intricacies of the physical world, the Dzhanibekov Effect serves as a reminder of the wonders that await us in our quest for understanding.

The video

References

1. Landau, L. D., & Lifshitz, E. M. (1976). Mechanics (3rd ed.). Pergamon Press.
2. Poinsot, L. (1851). Théorie nouvelle de la rotation des corps. Bachelier.
3. Tao, T. (2011). The Euler equations of motion. In An Epsilon of Room, II: Pages from Year Three of a Mathematical Blog. American Mathematical Society.
4. Veritasium. (2019). The Bizarre Behavior of Rotating Bodies, Explained. [Video]. YouTube.